The Law of Cosines (Cosine Rule)

The law of cosines is used to finding missing sides and angles of triangles.

 

Unlike the law of sines which is only useful for traingles in the form AAS, ASA, and SSA, this rule is used for the forms SSS and SAS

More information on types of triangles.

In fact, the pythagorean theorem is actually a special case of the cosine rule where the angle is 90 degrees and the form is SSS. Amazing!

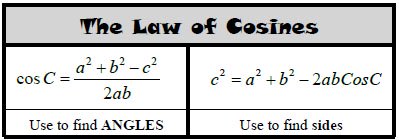
The Cosine Rule : c2 = a2 + b2 = 2abcosC

Before you attempt to use the formula shown above, please be sure that you understand the basics of trigonometry first - please be certain you are able to:

  • Evaluate the cosine of an angle using the cos function.
  • Find measures of angles using the inverse cosine function: cos-1
  • Understand the naming conventions for triangles (see below).
Naming Conventions for Sides and Angles of a Triangle

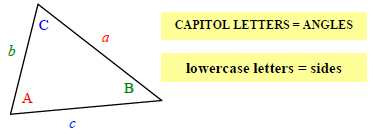
First, you must understand what the letters a, b, c and A, B, C represent in the formula. The corresponding letters (a & A, b & B, c & C) represent the side and the angle across from it. Please see the figure below.

Note: the letters used are arbitrary. As long as you match the side with the angle across from it, the formula will work!

The Law of Cosines (also known as the Cosine Rule)

   

The formula is listed in two forms: the standard form (used for sides) and a derivative of the standard form which makes it easier to colve for angles. Remember, to solve for an angle, you will eventually need to use the inverse function!

Examples Using the Cosine Rule
     
Example to find an Angle   Example to find a Side
 
     

Additional Information

Back to the top of the law of cosines

Back to trigonometry main page

Visit the GradeA homepage

    

 

 
Untitled Document

Stay connected

     
.


Home │ Site Search │ Math Help Blog │ Help Keep GradeA Free


Written by Team GradeAmathhelp.com, all rights reserved.